Lusana.ru - онлайн презентации
Добавь и Заработай

Презентация - Let’s start with what you know

Нужно больше вариантов? Смотреть похожие
Нажмите для полного просмотра
Let’s start with what you know
Распечатать
  • Уникальность: 99%
  • Слайдов: 80
  • Просмотров: 269
  • Скачиваний: 61
  • Размер: 4.37 MB
  • Онлайн: Да
  • Формат: ppt / pptx
В закладки
Оцени!
  Помогли? Поделись!
Содержание ▼
Читать онлайн!
Похожие презентации

Слайды и текст этой онлайн презентации

Слайд 1

Let’s start with what you know, слайд 1
Let’s start with what you know…
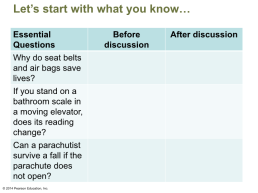
Essential Questions.Before discussion.After discussion
Why do seat belts and air bags save lives?..
If you stand on a bathroom scale in a moving elevator, does its reading change?..
Can a parachutist survive a fall if the parachute does not open?..
© 2014 Pearson Education, Inc.

Слайд 2

Let’s start with what you know, слайд 2
4 Things you know about forces
FORCES

Слайд 3

Let’s start with what you know, слайд 3
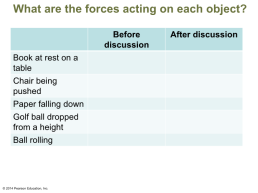
What are the forces acting on each object?
.Before discussion.After discussion
Book at rest on a table..
Chair being pushed..
Paper falling down..
Golf ball dropped from a height..
Ball rolling..
© 2014 Pearson Education, Inc.

Слайд 4

Let’s start with what you know, слайд 4
A push or a pull resulting from the object’s interaction with another object
FORCES
move
change direction
stop
SI Unit: Newtons (N)

Слайд 5

Let’s start with what you know, слайд 5
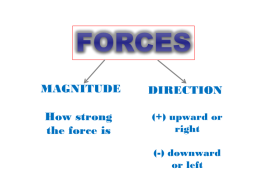
FORCES
MAGNITUDE
DIRECTION
How strong the force is
(+) upward or right (-) downward or left

Слайд 6

Let’s start with what you know, слайд 6
Forces
There is a good chance that 2 forces can be acting on an object at any one time

Слайд 7

Let’s start with what you know, слайд 7
INTERACTIONS
We choose one particular object for analysis; this object is called the system. (Ex. BOOK ON THE TABLE) All objects not part of the system can interact with it (touch it, pull it, and push it) and are in the system's environment. Interactions between the system object and objects in the environment are called external interactions. External interactions can affect the motion of the system.
© 2014 Pearson Education, Inc.

Слайд 8

Let’s start with what you know, слайд 8
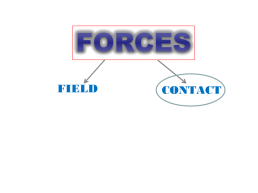
FORCES
FIELD
CONTACT

Слайд 9

Let’s start with what you know, слайд 9
Objects can interact directly (CONTACT), when they touch each other—for example, in a push or a pull. Objects can interact at a distance (FIELD)—for example, when a magnet attracts or repels another magnet without touching it.
© 2014 Pearson Education, Inc.

Слайд 10

Let’s start with what you know, слайд 10
CONTACT FORCES
AIR RESISTANCE ()
Acts on an object as it travels through air
Opposite the direction of motion

Слайд 11

Let’s start with what you know, слайд 11
CONTACT FORCES
FRICTION ()
Results from 2 surfaces being pressed together
Opposite the direction of motion

Слайд 12

Let’s start with what you know, слайд 12
CONTACT FORCES
NORMAL FORCE ()
support force exerted upon an object that is in contact with another stable object
Perpendicular to and away from the surface

Слайд 13

Let’s start with what you know, слайд 13
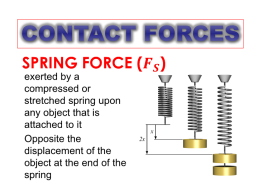
CONTACT FORCES
SPRING FORCE ()
exerted by a compressed or stretched spring upon any object that is attached to it
Opposite the displacement of the object at the end of the spring

Слайд 14

Let’s start with what you know, слайд 14
CONTACT FORCES
TENSION ()
transmitted through a string, rope, cable or wire when it is pulled tight by forces acting from opposite ends

Слайд 15

Let’s start with what you know, слайд 15
CONTACT FORCES
APPLIED FORCE ()
applied to an object by a person or another object

Слайд 16

Let’s start with what you know, слайд 16
FIELD FORCES
GRAVITATIONAL FORCE ()
All objects upon earth experience a force of gravity that is directed "downward" towards the center of the earth

Слайд 17

Let’s start with what you know, слайд 17
Mass vs. Weight
MASS
WEIGHT
Gravitational pull
Amount of matter in an object
SI Unit: Newtons
SI Unit: kilogram

Слайд 18

Let’s start with what you know, слайд 18
Mass vs. Weight

Слайд 19

Let’s start with what you know, слайд 19

Слайд 20

Let’s start with what you know, слайд 20
Balanced forces …constant velocity (a = 0) …at rest; motionless …vertical forces are equal …horizontal forces are equal

Слайд 21

Let’s start with what you know, слайд 21
Unbalanced forces …a is not equal to zero …vertical forces are not equal; and/or …horizontal forces are not equal

Слайд 22

Let’s start with what you know, слайд 22
Unbalanced Forces
If the forces acting on an object are not balanced then the object with either: Speed up Slow down Change direction Change its shape

Слайд 23

Let’s start with what you know, слайд 23
FREE BODY DIAGRAM
used to show the relative magnitude and direction of all forces acting upon an object in a given situation.

Слайд 24

Let’s start with what you know, слайд 24
Force diagrams
Used with the point-like model The system object is represented by a dot. Arrows used to represent the forces Length of the arrow relates to the strength of the force. Direction the arrow points relates to the direction in which the force is exerted on the system object. Includes forces exerted on the system object Shows the forces at a single instant
© 2014 Pearson Education, Inc.

Слайд 25

Let’s start with what you know, слайд 25
FREE BODY DIAGRAM
An egg is free-falling from a nest in a tree. Neglect air resistance. Diagram the forces acting on the egg as it is falling.

Слайд 26

Let’s start with what you know, слайд 26
FREE BODY DIAGRAM
A rightward force is applied to a book in order to move it across a desk with a rightward acceleration. Consider frictional forces. Neglect air resistance. Diagram the forces acting on the book.

Слайд 27

Let’s start with what you know, слайд 27
FREE BODY DIAGRAM
A rightward force is applied to a book in order to move it across a desk at constant velocity. Consider frictional forces. Neglect air resistance. Diagram the forces acting on the book

Слайд 28

Let’s start with what you know, слайд 28
FREE BODY DIAGRAM
A college student rests a backpack upon his shoulder. The pack is suspended motionless by one strap from one shoulder. Diagram the vertical forces acting on the backpack.

Слайд 29

Let’s start with what you know, слайд 29
FREE BODY DIAGRAM
A skydiver is descending with a constant velocity. Consider air resistance. Diagram the forces acting upon the skydiver.

Слайд 30

Let’s start with what you know, слайд 30
FREE BODY DIAGRAM
A car is coasting to the right and slowing down. Diagram the forces acting upon the car.

Слайд 31

Let’s start with what you know, слайд 31
Choosing a system to describe interactions (Cont'd)
© 2014 Pearson Education, Inc.

Слайд 32

Let’s start with what you know, слайд 32
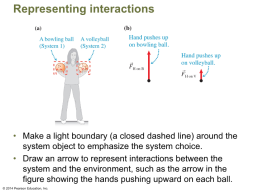
Representing interactions
Make a light boundary (a closed dashed line) around the system object to emphasize the system choice. Draw an arrow to represent interactions between the system and the environment, such as the arrow in the figure showing the hands pushing upward on each ball.
© 2014 Pearson Education, Inc.

Слайд 33

Let’s start with what you know, слайд 33
Drawing force diagrams
Sketch the situation. Circle the system. Identify external interactions. Place a dot at the side of the sketch representing the system object. Draw force arrows to represent the external interactions. Label the forces with a subscript containing two elements.
© 2014 Pearson Education, Inc.

Слайд 34

Let’s start with what you know, слайд 34
Constructing force diagrams
Example: a rock sinking into sand
© 2014 Pearson Education, Inc.

Слайд 35

Let’s start with what you know, слайд 35
Normal forces
Perpendicular touching forces are called normal forces. Normal forces are labeled using the letter N. Normal forces are contact forces (due to touching objects such as book "A" on book "B"). Normal forces are not always vertical as in the previous example.
© 2014 Pearson Education, Inc.

Слайд 36

Let’s start with what you know, слайд 36
Adding forces graphically
Draw the vectors head to tail. Draw the vector that goes from the tail of the first vector to the head of the second vector. This is the sum vector, also called the resultant vector. In this case this vector is the net force (it is not a new force, but rather the combined effect of all the forces being exerted on the object).
© 2014 Pearson Education, Inc.

Слайд 37

Let’s start with what you know, слайд 37
Sample Problem
You apply a 150 N force to lift a 100 N suitcase. Draw a free body diagram. Determine the resultant force in this situation graphically.
© 2014 Pearson Education, Inc.

Слайд 38

Let’s start with what you know, слайд 38
Adding forces graphically (Cont'd)
© 2014 Pearson Education, Inc.

Слайд 39

Let’s start with what you know, слайд 39
Patterns observed in the experiments
© 2014 Pearson Education, Inc.

Слайд 40

Let’s start with what you know, слайд 40
Observational experiments for a bowling ball rolling on a very hard, smooth surface
In all experiments, the vertical forces add to zero and cancel. We consider only forces exerted in the horizontal direction. In the first experiment, the sum of the forces exerted on the ball is zero. The ball's velocity remains constant. When the ruler pushes the ball, the velocity change arrow points in the same direction as the sum of the forces.
© 2014 Pearson Education, Inc.

Слайд 41

Let’s start with what you know, слайд 41
Testing possible relationships between force and motion
Two patterns are commonly proposed: The sum of the forces exerted is in the same direction as the velocity of the system object. The sum of the forces exerted is in the same direction as the change in velocity of the system object. We must do testing experiments to determine which pattern is consistent with the relationship between force and motion.
© 2014 Pearson Education, Inc.

Слайд 42

Let’s start with what you know, слайд 42
Testing possible relationships between force and motion
Two possible relationships: The sum of forces is in the same direction as the velocity. The sum of forces is in the same direction as the change in velocity. Use each relationship to predict the outcome of testing experiments. Perform the experiments and compare the outcomes with the predictions. From this comparison, decide whether we can reject one or both of the relationships.
© 2014 Pearson Education, Inc.

Слайд 43

Let’s start with what you know, слайд 43
Relating forces and motion
© 2014 Pearson Education, Inc.

Слайд 44

Let’s start with what you know, слайд 44
Reasoning without mathematical equations
Motion and force diagrams and the rule relating motion and force can be used to reason qualitatively about physical processes: To determine the relative magnitudes of forces if you have information about motion To estimate velocity changes if you have information about forces Make sure the unknown representation is consistent with the known representation.
© 2014 Pearson Education, Inc.

Слайд 45

Let’s start with what you know, слайд 45
Inertial reference frame
An inertial reference frame is one in which an observer: Sees that the velocity of the system object does not change if no other objects exert forces on it or Sees no change in the velocity if the sum of all forces exerted on the system object is zero In noninertial reference frames, the velocity of the system object can change even though the sum of forces exerted on it is zero.
© 2014 Pearson Education, Inc.

Слайд 46

Let’s start with what you know, слайд 46
Inertial reference frame
A passenger in a car or train that is speeding up or slowing down with respect to Earth is an observer in a noninertial reference frame. When you are in a car that stops abruptly, your body jerks forward, yet nothing is pushing you forward. Observers in noninertial reference frames cannot explain the changes in velocity of objects by considering the forces exerted on them by other objects.
© 2014 Pearson Education, Inc.

Слайд 47

Let’s start with what you know, слайд 47
Newton's first law of motion
For an observer in an inertial reference frame, the object continues moving at constant velocity (including remaining at rest): When no other objects exert forces on an system object or When the forces exerted on the object add to zero Inertia is the phenomenon in which an object continues to move at constant velocity when the sum of the forces exerted on it by other objects is zero.
© 2014 Pearson Education, Inc.

Слайд 48

Let’s start with what you know, слайд 48
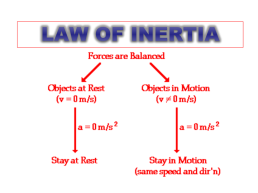
LAW OF INERTIA
A body at rest remains at rest, or a body in motion remains in constant velocity unless acted upon by an unbalanced force.

Слайд 49

Let’s start with what you know, слайд 49
INERTIA
Tendency of a body to resist change in motion

Слайд 50

Let’s start with what you know, слайд 50
MASS AND INERTIA
Greater mass, greater inertia

Слайд 51

Let’s start with what you know, слайд 51
LAW OF INERTIA AND FOOTBALL

Слайд 52

Let’s start with what you know, слайд 52
MASS AND INERTIA
Peter is being chased through the woods by a bear that he was attempting to photograph. The enormous mass of the bear is extremely intimidating. Yet, if Peter makes a zigzag pattern through the woods, he will be able to use the large mass of the moose to his own advantage. Explain this in terms of inertia and Newton's first law of motion.

Слайд 53

Let’s start with what you know, слайд 53
LAW OF INERTIA AND DRIVING
Why is seatbelt considered a safety device?

Слайд 54

Let’s start with what you know, слайд 54
LAW OF INERTIA

Слайд 55

Let’s start with what you know, слайд 55
LAW OF INERTIA

Слайд 56

Let’s start with what you know, слайд 56
Newton's second law of motion
Observation experiments help us construct the following relationship between the sum of forces on a system object and the system object's motion: The symbol α means "is proportional to." For example, if the sum of the forces doubles, then the acceleration doubles.
© 2014 Pearson Education, Inc.

Слайд 57

Let’s start with what you know, слайд 57
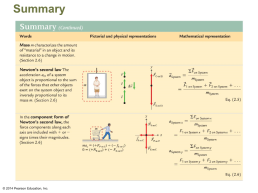
Mass
Mass is a measure of the amount of matter. Mass is represented by the symbol m. To measure mass quantitatively, you first define a standard unit of mass. The SI standard unit of mass is the kilogram (kg).
© 2014 Pearson Education, Inc.

Слайд 58

Let’s start with what you know, слайд 58
Mass
Mass characterizes the amount of matter in an object. When the same unbalanced force is exerted on two objects, the object with greater mass has a smaller acceleration. Mass is a scalar quantity, and masses add as scalars.
© 2014 Pearson Education, Inc.

Слайд 59

Let’s start with what you know, слайд 59
Newton's second law of motion
Observation experiments help us construct the following relationship for the proportionality between the acceleration of a system object and the system object's mass:
© 2014 Pearson Education, Inc.

Слайд 60

Let’s start with what you know, слайд 60
Newton's second law of motion
Combining the results of our observational experiment findings, we have: Force is a ubiquitous quantity so it has a unit defined for it called a newton (N). A force of 1 newton (1 N) causes an object with a mass of 1 kg to accelerate at 1 m/s2.
© 2014 Pearson Education, Inc.

Слайд 61

Let’s start with what you know, слайд 61
Newton's second law of motion
"Vector sum of the forces" means we cannot add the forces as numbers; the directions of the vectors affect the magnitude of the vector sum.
© 2014 Pearson Education, Inc.

Слайд 62

Let’s start with what you know, слайд 62
Making sense of Newton's second law
The equation we deduced for Newton's second law is: If the mass is infinitely large, the acceleration is zero. If the mass is zero, the acceleration is infinitely large. Both of these extreme cases make sense.
© 2014 Pearson Education, Inc.

Слайд 63

Let’s start with what you know, слайд 63
Cause-effect relationships
The equation we deduced for Newton's second law is: The right side of the equation (the sum of the forces being exerted on the system) is the cause of the effect (the system's acceleration) on the left side.
© 2014 Pearson Education, Inc.

Слайд 64

Let’s start with what you know, слайд 64
Operational definition versus cause-effect
The equal sign in Newton's second law does not imply the same thing as the equal sign used for the definition of acceleration. is a cause-effect relationship: why the acceleration occurs. is an operational definition: how to determine a quantity by defining it in terms of another quantity (but does not tell the reason for the acceleration!).
© 2014 Pearson Education, Inc.

Слайд 65

Let’s start with what you know, слайд 65
Force components used for forces along one axis
Our equation for Newton's second law can be written in vector component form. For example, in the x-direction we have: Identify the positive direction of the axis. Find the components of all the forces being exerted on the system. Forces that point in the positive direction have a positive component; forces that point in the negative direction have a negative component.
© 2014 Pearson Education, Inc.

Слайд 66

Let’s start with what you know, слайд 66
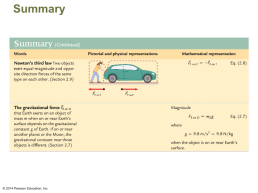
Gravitational force law
Objects falling in a vacuum (for instance, a tube with the air removed) show that all objects fall straight down with the same acceleration. This acceleration has a magnitude of 9.8 m/s2. Earth (E) exerts the only force on the falling object (O) (in a vacuum). FE on Oy = mOaOy = mO(9.8 m/s2) We define g such that:
© 2014 Pearson Education, Inc.

Слайд 67

Let’s start with what you know, слайд 67
Gravitational force
© 2014 Pearson Education, Inc.

Слайд 68

Let’s start with what you know, слайд 68
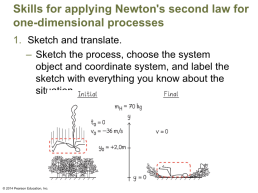
Skills for applying Newton's second law for one-dimensional processes
Sketch and translate. Sketch the process, choose the system object and coordinate system, and label the sketch with everything you know about the situation.
© 2014 Pearson Education, Inc.

Слайд 69

Let’s start with what you know, слайд 69
Skills for applying Newton's second law for one-dimensional processes (Cont'd)
Simplify and diagram. Make appropriate simplifying assumptions and represent the process with a motion diagram and/or a force diagram.
© 2014 Pearson Education, Inc.

Слайд 70

Let’s start with what you know, слайд 70
Skills for applying Newton's second law for one-dimensional processes (Cont'd)
Represent mathematically. Convert the representations into quantitative mathematical descriptions using kinematics and Newton's second law. Solve and evaluate. Substitute the known values and solve, and then evaluate your work to see if it is reasonable. Check whether all representations are consistent.
© 2014 Pearson Education, Inc.

Слайд 71

Let’s start with what you know, слайд 71
Weight
The weight of the object on a planet is the force that the planet exerts on the object. In everyday language, the normal force that a scale exerts on you (which balances the downward force you exert on it) is your weight. We will not use the term "weight of an object" because it implies that weight is a property of the object rather than an interaction between two objects.
© 2014 Pearson Education, Inc.

Слайд 72

Let’s start with what you know, слайд 72
Forces come in pairs
Suppose you wear rollerblades and push abruptly on a wheeled cart loaded with a heavy box. If you and the cart are on a hard smooth floor, the cart starts moving away (it accelerates), and you also start to move and accelerate in the opposite direction. You exerted a force on the cart and the cart exerted a force on you. Because the accelerations were in opposite directions, the forces must point in opposite directions.
© 2014 Pearson Education, Inc.

Слайд 73

Let’s start with what you know, слайд 73
Testing experiment: Newton's third law of motion
Attach one spring scale to a hook on the wall and pull on its other end with a second spring scale. If the hypothesis is correct, then the scale you pull should have the same reading as the scale fixed to the wall. You find that the scales have the same readings. If you reverse the scales and repeat the experiment, you find they always have the same readings.
© 2014 Pearson Education, Inc.

Слайд 74

Let’s start with what you know, слайд 74
Newton's third law of motion
When two objects interact, object 1 exerts a force on object 2. Object 2 in turn exerts an equal-magnitude, oppositely directed force on object 1. These forces are exerted on different objects and cannot be added to find the sum of the forces exerted on one object.
© 2014 Pearson Education, Inc.

Слайд 75

Let’s start with what you know, слайд 75
Tips for Newton's third law of motion
The forces in Newton's third law are exerted on two different objects. This means that the two forces will never appear on the same force diagram. Also, they should not be added together to find the sum of the forces. You have to choose the system object and consider only the forces exerted on it!
© 2014 Pearson Education, Inc.

Слайд 76

Let’s start with what you know, слайд 76
Putting it all together: Air bags
An air bag is like a balloon made of heavy-walled material that is packed in a small box. It is designed to deploy when a car has an acceleration of 10 g or more (~98 m/s2). The bag: Spreads out the force that stops the person over a larger area of the body Increases the stopping distance, consequently reducing the average force to stop the driver
© 2014 Pearson Education, Inc.

Слайд 77

Let’s start with what you know, слайд 77
Summary
© 2014 Pearson Education, Inc.

Слайд 78

Let’s start with what you know, слайд 78
Summary
© 2014 Pearson Education, Inc.

Слайд 79

Let’s start with what you know, слайд 79
Summary
© 2014 Pearson Education, Inc.

Слайд 80

Let’s start with what you know, слайд 80
Summary
© 2014 Pearson Education, Inc.
^ Наверх
X
Благодарим за оценку!

Мы будем признательны, если Вы так же поделитесь этой презентацией со своими друзьями и подписчиками.